Degenerate Conic Sections

If b2 4ac 0 the equation represents a.

Degenerate conic sections. Like other conic sections all degenerate conic sections have equations of the form a x 2 b xy c y 2 d x e y f 0. Two intersecting lines such as x 2 y 2 0 x y x y 0 displaystyle x 2 y 2 0 leftrightarrow. Two parallel lines such as x 2 1 0 x 1 x 1 0 displaystyle x 2 1 0 leftrightarrow x 1.

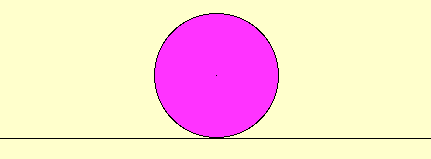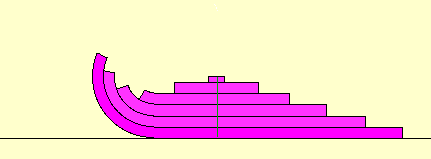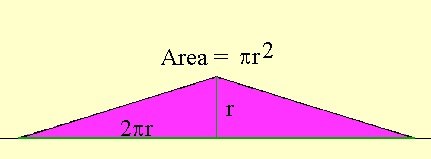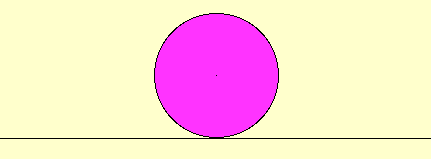
The types of conic sections are circles ellipses hyperbolas and parabolas. A conic section which does not fit the standard form of equation. Degenerate conic sections plane figures that can be obtained by the intersection of a double cone with a plane passing through the apex.

If the plane passes through the apex of the cone where the two halves of the cone meet you end up with a point a line or two intersecting lines depending on the angle of the plane. Back miscellaneous mathematics mathematics contents index home. These take the form of points and lines.

If b2 4ac 0 the equation represents an ellipse. Those are typically what are considered degenerate conic sections. A conic section is a section of a cone.

These include a point a line and intersecting lines. Each conic section also has a degenerate form. If a c and b 0 the equation represents a circle which is a.

If a c and b 0 the equation represents a circle which is a special case of an ellipse. A degenerate real conic may be. A double line multiplicity 2.